Math 560/561 - Applied Math I/II *
![]()
Math 560/561 - Applied Math I/II *
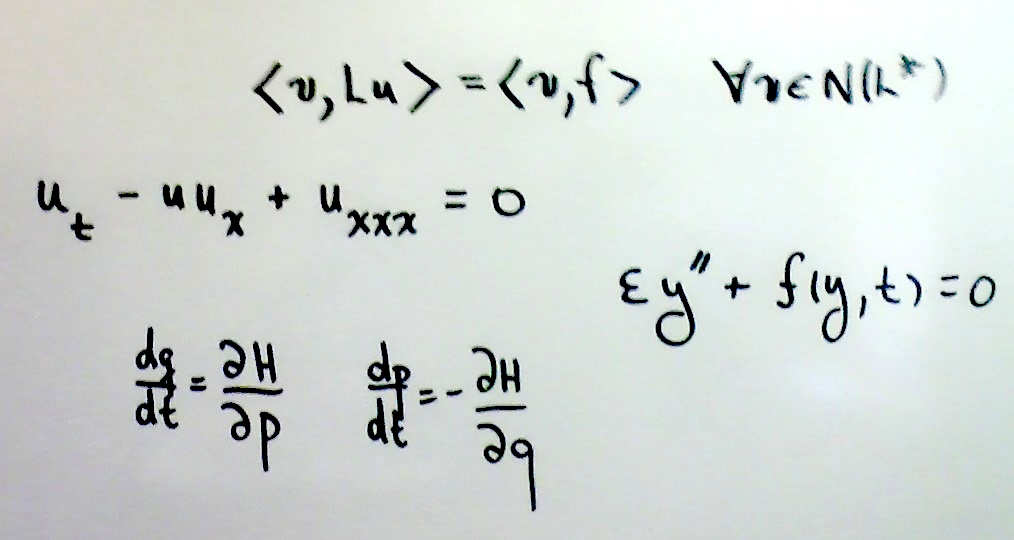
![]()
| Textbook | Principles of Applied Mathematics (1rst or 2nd ed.), James Keener (not required but highly recommended) | ||||||||||||||||||||
| Instructor | Mark Pernarowski | ||||||||||||||||||||
| Grading | Your grade will be based strictly on homework assignments. The raw score for each
assignment will vary. If P is the percentage of the sum of all the raw scores then
your letter grade will be determined from:
|
||||||||||||||||||||
Assignments must be written in a clear and logical fashion. |
|||||||||||||||||||||
![]()
| Homework | Homework 1 :Inner product spaces, diagonalization, Fredholm applications, Least Sq. | Due: Thursday, Sept 22 |
| Homework 2: Operator boundedness, eigenfunctions, adjoint calculations | Due: Thursday, Oct 13 | |
| Homework 3: Operators, Fredholm alternative and Distributions | Due: Thursday, Nov 3-7 | |
| Homework 4: Green's functions and Sturm Liouville | Due: Nov 17-22 | |
| Homework5: Euler Lagrange Eqns, Natural BC, Extrema, Geodesics | Due: Thursday, Feb 2 | |
|
|
Due: Thursday, Feb 23 | |
|
|
Due: Thursday, Mar 30 | |
|
|
Due: Thursday, Apr 20 |
![]()
![]()
| Supplemental | Operator Theory Summary Sheet and Compact Operators | |
| Boundary Value Problems - 2nd order | ||
| Integral Equation examples | ||
| Functional Analysis - Bounded Operators | ||
| Functional Analysis - Fredholm Alternative | ||
| Functional Analysis - Mixed with Integral equations | ||
|
|
Upon completion of the course students will have an understanding of the following:Inner product spaces: Bases, coordinates, Gram Schmidt orthogonalization, Least squares approximations Matrices: adjoints, diagonalization, maximum principle, projections, Least squares solutions, singular value decomposition, normal equations and the Fredholm alternative Introductory theory of Hilbert spaces including an overview of the properties of bounded, unbounded, and compact operators and their adjoints. Completeness and spectral properties of self adjoint operators. Fredholm alternative and applications. Operator inverses. Introduction to distributions on test function spaces and their properties. Green's functions and Sturm Liouville theory for Boundary Value Problems. Calculus of Variations introduction: functionals, admissible sets, natural boundary conditions for Lagrangians of single and several dependent variables. Euler Lagrange equation derivations Intermediate Calculus of Variation topics: geodesics, isoperimetric problems, transversality, Lagrangians with several independent variables, convexity and sufficiency conditions for minima. Lagrangian and Hamiltonian mechanics and the principle of Least action. Regular perturbation theory for algebraic and differential systems. Implicit function Theorem. Asymptotic expansions and series with applications to root approximation. Matching Theory for asymptotic expansions, overlap domains, intermediate variables. Matched asymptotic expansions for boundary value problems. Perturbation techniques for oscillatory systems: Multiple scales approaches with different temporal straining, the method of averaging and adiabatic invariants. |
|
